Linear Inequalities

An inequality is a statement that one quantity is greater than or less than another quantity as a comparison of one quantity to another.

Linear Inequalities are first-degree, meaning the variable in a quantity has an exponent of 1.

The math symbols to state an inequality are:

> (is Greater Than)

The quantity to the left is larger than the quantity to the right of this symbol.

< (is Less Than)

The quantity to the left is smaller than the quantity to the right of this symbol.

≥ (is Greater Than or Equal)

The quantity to the left is larger than or equal to the quantity to the right of this symbol.

≤ (is Less Than or Equal)

The quantity to the left is smaller than or equal to the quantity to the right of this symbol.

A Linear Inequality example is:

3X − 2 > 2X + 1

If X = 5 then 13 > 11 and 5 is a value that correctly satisfies this inequality.

If X = 2 then 4 > 5 is incorrect, 2 does not correctly satisfy the inequality.

There are other values (of X) that would correctly solve this inequality.

Solving an inequality means finding its solution set, and two inequalities that have the same (identical) solution set are equivalent. Similar to equations (=, Equals) solving Linear Inequalities is by finding equivalent inequalities from which solutions can be easily seen. To accomplish this use the following math properties to reduce an inequality to a simple equivalent inequality:

Substitution Property of Inequalities

Substituting one expression for an equal expression is equivalent to the original inequality:

7X − 5X < 6 is equivalent to 2X < 6 and X < 3

It can now be easily seen that any value of X less than 3 is a solution of the inequality. We would write the solution set using set notation as:

{X:X < 3} Read as: X, such that X, is less than 3.

Addition and Subtraction Property of Inequalities

Adding the same quantity to both sides of an inequality is equivalent to the original inequality:

X − 6 < 12 is the same as X < 18

Subtracting the same quantity to both sides of an inequality is equivalent to the original inequality:

X + 6 > 12 is the same as X > 6

Multiplication and Division Properties for Inequalities

Property 1:

When we multiply or divide both sides of an inequality by the same positive quantity it is equivalent to the original quantity:

¼ X > 7 is the same as X > 28

4X < 16 is the same as X < 4

Property 2:

When we multiply or divide both sides of an inequality by a negative number and reverse the sense of the inequality it is equivalent to the original inequality:

−5 < 3 is the same as 5 > −3

−3X > −27 is the same as 3X < 27 and X < 9

X/−3 < −9 is the same as X > 27 And has a solution set of: {X:X > 27}

However, we cannot multiply or divide both sides of an inequality by zero, the result will not be equivalent to the original inequality.

Inequality problems solved for you:

2(X − 4) < (X − 3)/3 =

6(X − 4) < X − 3 =

6X − 24 < X − 3 =

6X − X < −3 + 24 =

5X < 21 =

X < 21/5 so that {X:X < 21/5}

3(X + 2 ) > X/4 + 2 =

3X + 6 > X/4 + 2 =

12X + 24 > X + 8 =

11X > −16 =

X > −16/11 so that {X:X > −16/11}

Compound Inequalities

Are inequalities with more than one math inequality symbol.

−2 < X < 4 is a Compound Inequality, and represents an open interval if graphed on a number line.

The end-points, −2 and 4, are not included in the solution set:

Open Interval Compound Inequality on Number Line.

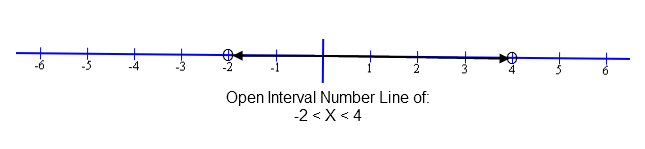
Just by changing an inequality math symbol we create a different line segment on a number line.

−2 < X ≤ 4 is a Compound Inequality, and represents a half-open interval if graphed on a number line.

The end-point, −2, is not included in the solution set while 4 is part of the solution set:

One Math Symbol is ≥ or ≤ of an Inequality that graphs a Half Open Interval line segment.

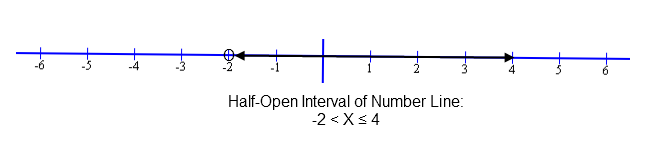
If all math symbols of the inequality are ≥ or ≤ we would not attach small circles at line segment end points.

−5 ≤ X ≤ 12 is a Compound Inequality, and represents a closed interval if graphed on a number line.

The end-points, −5 and 12, are both included in the solution set.

To solve Compound Inequalities we split the inequality into separate simple inequalities.

2X − 4 < 3X ≤ 2X + 3

is a Compound Inequality, and can be easily separated into two simple inequalities:

2X − 4 < 3X =

−4 < X

And

3X ≤ 2X + 3 =

X ≤ 3

The final step is to put the simple inequalities together:

The solution, −4 < X ≤ 3, graphs as a half-open interval.

One end point, −4, is not included in the solution set

while the other end point, 3, is included in the solution set:

{X:X > −4} ∩ {X:X ≤ 3}

Top of Page

About Privacy